You’ve probably heard this before: Why do we need to know this? While some math concepts might truly be a touch irrelevant to the everyday lives of our students, they still need to achieve these skills to move on to the next grade. Learning a skill for the sake of learning another skill, however, isn’t really the point of your math classroom. As math teachers, you are teaching students to analyze and solve complex problems with precision. You are teaching students to become critical thinkers that can confidently approach a wide range of tasks. These are the mathematical mindsets that students take with them far beyond the reaches of your classroom walls.
Where content and procedural skills are often grounded in Common Core Standards for Mathematical Content, these mathematical mindsets live within the Common Core Standards for Mathematical Practice. Within the Fishtank Math curriculum, we have connected the eight Mathematical Practice (MP) Standards to our content to help teachers identify and take advantage of opportunities to focus on building these mindsets with students. Even though all eight of the MP Standards are important for students to develop, we have chosen to focus this blog post on three of the MP Standards that we believe are highly impactful for students in grades 3–8.
Make Sense of Problems and Persevere in Solving Them: Mathematical Practice Standard 1
Making sense of problems and persevering in solving them is probably the most important skill we can teach students to apply both inside and outside of the classroom. As students progress throughout their education, they will be faced with content that is more challenging and may have less support from teachers, and no support when they face standardized exams.
Teaching our students to analyze a problem, figure out what they are being asked to do, and create a plan to bridge what they already know from the problem with what they want to know is a skill they can apply to any math problem they face. Beyond math, this same approach of analyzing a situation, determining the end goal, and making a plan to get there applies to any obstacle our students may face in their future. Perseverance is a helpful skill in the classroom and an often necessary skill outside of the classroom.
When considering how to help students build their ability to make sense of problems and persevere in solving them, it is important to model the metacognitive strategies we want students to use. Let’s explore this in practice. Below is an Anchor Problem from Lesson 20 of our 8th Grade Transformations and Angle Relationships unit.
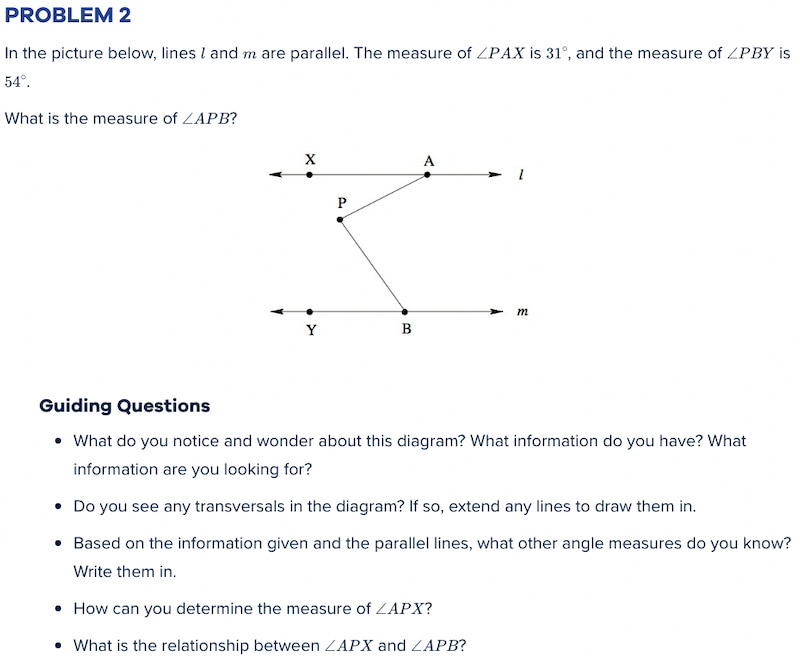
At first, this problem might seem complex and intimidating to students. However, we want our students to be able to confidently tackle challenging problems. In this example, we can help students make sense of the problem and begin the process of solving. The outlined Guiding Questions provide you with a jumping off point for eliciting student thinking. You might start by asking students what they notice about the diagram, what information they have access to, and how the relationships shown might indicate the necessary steps to solve. As you guide students through this process, they are likely to realize that they can find the measure of the angle by extending the transversals and applying their previously developed knowledge of triangles and alternate interior angles.
There are multiple concepts students must draw upon to solve this problem, but by teaching students to critically analyze the information they are given and the question they are being asked to address, we set them up to successfully solve.
Construct Viable Arguments and Critique the Reasoning of Others: Mathematical Practice Standard 3
In many cases, getting the correct answer isn’t enough. We want our students to understand the why behind the work they do. By teaching students to construct viable arguments that defend their thinking, we teach them to focus on why their answers make sense. This allows students to transfer their thinking beyond a single problem as they think critically about their solving processes. When they learn to critique the reasoning of others, they build effective communication skills and develop the ability to analyze different types of thinking.
These skills are helpful in the math classroom as students gain an understanding of the different approaches to problem solving and the ways in which they can justify their reasoning. Outside of the classroom, students bridge these skills to any number of issues they encounter that require them to work with others. By teaching students to construct viable arguments and critique the reasoning of others, we set them up to successfully engage as part of a group or team in an educational, professional, or personal setting.
When considering what teaching this skill looks like in practice, it is important to plan opportunities for students to defend their thinking aloud and respond to one another. Let’s explore an example from Lesson 8 of our 4th Grade Decimal Fractions unit.

This problem asks students not only which option they would rather have, but why they would choose it. You might plan to have students debate each of the three scenarios so that students can practice defending their answer, and have the opportunity to critique the reasoning of others. You can push students to prove their reasoning with models or mathematical expressions and encourage them to use academic language while debating. This problem creates a rich opportunity for students to explore decimal numbers while also encouraging students to develop their ability to explain their thinking clearly. Teaching students to communicate their ideas and respectfully critique the thinking of others is a mathematical skill they can apply anywhere.
Attend to Precision: Mathematical Practice Standard 6
Attending to precision is incredibly important in math class as there are often so many small things (a negative sign, an inequality symbol, a unit) that students need to pay close attention to when solving. Many students can get frustrated when they have worked through all the steps of a complicated problem only to realize that they have misread a number in the problem or incorrectly set up their equation for solving. By teaching students to attend to precision, we teach them the importance of slowing down, digesting the information before them, and expressing numerical solutions with a degree of precision appropriate for the context of the problem.
Similarly, outside of our specific 3–8 math classrooms, students are often faced with tasks that require their precision. Beyond the ability to compute precisely, our students also benefit from the ability to determine what level of precision is necessary for a given task: Sometimes it is more efficient to use estimation rather than a precise calculation.
While it’s easy to say it’s important to attend to precision, perhaps the more interesting questions are why and when it’s important to be precise. In practice, these are two broad levels at which we can teach our students to attend to precision. First, we focus on the specific details we want our students to recognize and address in their communication of mathematical computations. Second, we help our students understand what level of precision is necessary based on the context of a given problem. Both of these skills ensure our students are equipped to tackle challenging content with confidence and accuracy.
Let’s look at an example that focuses on the need for students to communicate their mathematical thinking precisely. This Anchor Problem comes from Lesson 7 of the 6th Grade Equations and Inequalities unit.

In this example, students need to be precise in their communication of their solving process. First, students need to recognize that they have to clearly define a variable. Some students may say that their variable represents “Sierra” or “Sierra’s Walk”. Neither of these are very clear! Here, attending to precision begins with defining the variable as “the distance of Sierra’s morning walk in miles”. Students must use precision here to show that they are solving for a distance in miles or their communication of their answer won’t actually align with the question. Beyond defining the variable, students must attend to precision as they navigate between one expression that represents a single day’s distance and one expression that represents one week’s distance. By teaching students the importance of communicating their solving processes and creating representations of their thinking precisely, we help them understand the importance of each detail within their work. Not only does this support individual student’s comprehension, but it also allows others who look at the work to understand that student’s thought process.
Next, let’s look at an example that helps students understand what level of precision is required to most efficiently and appropriately address a problem. This Anchor Task comes from Lesson 12 of the 4th Grade Place Value, Rounding, Addition, and Subtraction Unit.

In this example, students explore the potential advantages and disadvantages of rounding to various places for a given context. By this point in the unit, students understand how to round and could therefore decide that they want to be as precise as possible and round to the nearest ten, hundred, or thousand like the problem suggests. However, what students come to realize is that rounding to the nearest thousand, while more precise than rounding to the nearest ten thousand or hundred thousand, would not be an appropriate strategy in this context since it would result in an underestimate so visitors might be left without a map! Instead, students learn to recognize that the goal of this problem is to generate an estimate that would provide enough maps for future visitors and maybe even some wiggle room in case there are even more visitors next year, and therefore being as precise as possible is not helpful. By teaching our students to assess the level of precision appropriate for a given situation, we help them see the bigger picture and more efficiently find a solution.
Ready to start teaching these mathematical mindsets in your own classroom? Dive into Fishtank Math today and explore the Expanded Assessment Package available to Fishtank Plus users for problems that are tagged for each of the Mathematical Practice Standards.
Rachel Fuhrman is the Curriculum Marketing Manager at Fishtank Learning. Before joining Fishtank Learning, Rachel spent 5 years as a Middle School Special Education Teacher in New Orleans, LA and Harlem, NY. Outside of the classroom, she has been a frequent contributor to multiple education blogs and focuses primarily on student engagement and instructional practice topics. Rachel earned both her Bachelor of Arts in Economics and her Master of Science in Educational Studies from The Johns Hopkins University.